IGNOU MEC-03/MEC-103
- Quantitative Methods,
Latest Solved Assignment
(July 2024 - January 2025)

Section A

Answer the following questions in about 700 words each. The word limits do not apply in case of numerical questions.

1. a. Consider the following utility maximization problem, in which the utility function of the consumer is given by U(x,y)=x0.5y0.5. The consumer income is I=100 and px=2 and py=3. Find the utility maximizing x and y when the budget constraint of the consumer may or may not bind. (Apply Kuhn-Tucker method)
b. Explain the theorem of second order optimum.

2. Given the input matrix and the final demand vector:

a) Explain the economic meaning of the elements 0.33,0 and 200
b) Explain the economic meaning of (if any) of the third column sum
c) Explain the economic meaning of (if any) of the third row sum
d) Write out the specific input-output matrix equation for this model
e) Find the solution output levels of the three industries using Cramer’s rule.

Section B

Answer the following questions in about 400 words each. The word limits do not apply in case of numerical questions.

3. Using Simplex method solve the problem:
Maximise 𝜋 = 6𝑥1 + 2𝑥2 + 5𝑥3

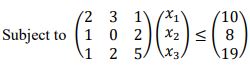
x1, x2, x3 ≥ 0

4. a.) A salesman has 50% chance of making a sale. If two customers enter the shop, what is the probability that the salesman will make a sale?
b.) If the events A and B are independent, then show that Ac, Bc, A and B are pair wise independent.

5. a) Discuss the nature of the following time path: 𝑦𝑡 = 3𝑡 + 1
b) Solve equation: 𝑦𝑡+1 −1/3𝑦𝑡 = 6 𝑓𝑜𝑟 𝑦0 = 1

6. Examine the following functions for maxima and minima:

a) 𝑧 = 2𝑥2 + 𝑥𝑦 + 4𝑦2 + 𝑥𝑧 + 𝑧2 + 2
b) 𝑧 = 𝑒2𝑥 − 𝑒𝑦 + 𝑒2𝑧 − 2(𝑥 + 𝑒) + 𝑦

7. Write short notes on following:

e) Euler’s theorem
f) Riemann Sum
g) Point of inflexion
h) Chi square test of goodness of fit

Section A

Answer the following questions in about 700 words each.

1) (i)What do you understand by the terms ‘test of significance’? Why do we need to test any distribution for significance? Explain the reasons.
ii) Discuss the concepts of confidence intervals and confidence limits with the help of an example.
(iii) Distinguish between one-tailed test and two-tailed test.

2) Consider the following case of constrained optimisation
U(x, y) = x2y and Px=2, Py=l. Total income, I=200.
where consumer’s utility (U) is dependent upon the two goods x and y. I is the total income of the consumer. The consumer spends all the income on spending over two goods x and y. Maximise the utility given the income constraint. Find out the optimal consumption bundle (x*, y*) and the maximum utility of the consumer.

Section B

Answer the following questions in about 400 words each. 

3) Explain the properties of set operations with examples.

4) What is a discontinuous function? Discuss the two types of discontinuous functions along with their diagrams.

5) Explain the concept of a stationary point and inflexion point. Is a stationary point always a point of inflexion? Why or why not?

6) Define normal distribution. Discuss the two parameters which are integral to its definition.

7) Write short notes on the following:

(i) Local maxima
(ii) Mapping and function
(iii) Biases in the survey
(iv) Finite and infinite sets

खंड - क

प्रत्येक प्रश्न का उत्तर लगभग 700 शब्दों में देना है। परिमाणात्मक प्रश्नों पर शब्द सीमा लागू नहीं होती ।

1. (क) निम्नलिखित उपयोगिता अधिकतमकरण समस्या पर विचार करें, जिसमें उपभोक्ता का उपयोगिता कार्य U(x,y) = x 0.5 y0.5 द्वारा दिया गया है। उपभोक्ता आय I = 100 है और px = 3 । जब उपभोक्ता की बजट बाधा बाध्य हो भी सकती है और नहीं भी, तो x तथा y को अधिकतम करने वाली उपयोगिता ज्ञात कीजिए। (कुछ टकर विधि लागू करें)
(ख) द्वितीय क्रम इष्टतम की प्रमेय की व्याख्या कीजिए ।

2. इनपुट मैट्रिक्स और अंतिम मांग वेक्टर को देखते हुए:

(क) 0.33.0 और 200 के अवयवों का आर्थिक अर्थ समझाइए
(ख) तीसरे स्तंभ योग के (यदि कोई हो) का आर्थिक अर्थ समझाइए
(ग) तीसरी पंक्ति के योग (यदि कोई हो) का आर्थिक अर्थ समझाइए
(घ) इस मॉडल के लिए विशिष्ट इनपुट-आउटपुट मैट्रिक्स समीकरण लिखें
(ड) क्रमर के नियम का उपयोग करके तीन उद्योगों के समाधान उत्पादन स्तर का पता लगाएं।

खंड - ख

प्रत्येक प्रश्न का उत्तर लगभग 500 शब्दों में देना है। परिमाणात्मक प्रश्नों पर शब्द सीमा लागू नहीं होती।

3. सरल (simplex) विधि का उपयोग करके समस्या का समाधान करे
भूयिष्ठ बनाएं π = 6x1 + 2x2 + 5x3

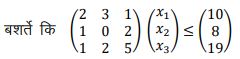
और 𝑥1,𝑥2, 𝑥3 ≥ 0

4. (क) एक विक्रेता के पास बिक्री करने का 50% मौका है। यदि दो ग्राहक दुकान में प्रवेश करते हैं. तो विक्रेता द्वारा बिक्री किए जाने की प्रायिकता क्या है?
(ख) यदि घटनाएं A और B स्वतंत्र हैं, तो दर्शाइए कि Ac, Bc, A और B युग्म वार स्वतंत्र हैं।

5. (क) निम्नलिखित समय पथ की प्रकृति पर चर्चा कीजिए: 𝑦𝑡 = 3 𝑡 + 1
(ख) समीकरण को हल करें: Yo=1 के लिए 𝑦𝑡+1 − 1/3 𝑦𝑡 = 6

6. नीचे दिए गए फलनों की भूयिष्ठकों अथवा अल्पिष्ठकों हेतु जांच कीजिए:

(क) z= 2𝑥 2 +𝑥𝑦 + 4𝑦 2 + 𝑥𝑧 + 𝑧 2 + 2
(ख) z= 𝑒 2𝑥 − 𝑒 𝑦 + 𝑒 2𝑧 − 2(𝑥 + 𝑒) + 𝑦

7. निम्नलिखित पर संक्षिप्त टिप्पणीयाँ लिखिए:

(क) यूलर प्रमेय
(ख) रीमन संकलन (Riemann Sum )
(ग) नीति परिवर्तन बिंदु (Inflexion Point)
(घ) उपयुक्तता के गुण की काई-वर्ग जाँच (Chi square Test of goodness of Fit )

भाग (क)

1. (i) 'सार्थकता सम्बन्धी परीक्षण से आप क्या समझते हैं? सार्थकता के लिए हमें किसी बंटन का परीक्षण करने की आवश्यकता क्यों है ? कारण स्पष्ट कीजिए।
(ii) एक उदाहरण की सहायता से गोपन अंतराल (कॉन्फिडेंसइंटरवल्स) और गोपन सीमान्त (कॉन्फिडेंसलिमिट्स) की अवधारणाओं पर चर्चा कीजिए ।
(iii) एक पुच्छ परीक्षण और दो पुच्छ परीक्षण में अंतर स्पष्ट कीजिए।

2. निबाधितइष्टतमीकरण के निम्नलिखित स्थिति पर विचार कीजिए:
U(x,y)=x2y और Px = 2, Py = 1, कुल आय, I = 200
जहां उपभोक्ता की उपयोगिता (U) दो वस्तुओं x और y पर निर्भर है। 1, उपभोक्ता की कुल आय है। उपभोक्ता पूरी आय को दोनों वस्तुओं x और y को खरीदने पर खर्च करता है।
आय निबाध को देखते हुए उपयोगिता को अधिकतम करें। इष्टतम उपभोग बंडल (x*, y *) और उपभोक्ता की अधिकतम उपयोगिता ज्ञात कीजिए।

भाग (ख)

3. समुच्चय संक्रियाओं के गुणों को उदाहरण सहित समझाइए ।

4. एक असतत फलन क्या है? दो प्रकार के असतत फलनों की उनके रेखाचित्रों के साथ चर्चा कीजिए ।

5. एक स्तब्ध (स्थिर) बिंदु और नति परिवर्तन बिंदु की अवधारणा को समझाइए | क्या एक स्तब्ध (स्थिर) बिंदु मेशा एक नति परिवर्तन बिंदु होता है? क्यों या क्यों नहीं?

6. प्रसामान्यबंटन (सामान्य वितरण) को परिभाषित कीजिए। उन दो मापदंडों पर चर्चा करें जो इसकी परिभाषा के अभिन्न अंग हैं।

7. निम्नलिखित पर संक्षिप्त टिप्पणी लिखिए:

i) स्थानिक भूयिष्ठिक
ii) प्रतिचित्रण और फलन
iii) सर्वेक्षण से पूर्वाग्रह
iv) परिमित और अपरिमित समुच्चय