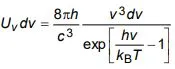PLEASE MATCH YOUR ASSIGNMENT QUESTIONS ACCORDING TO YOUR SESSION
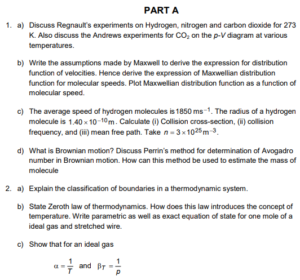
IGNOU BPHCT-135 (January 2025 – December 2025) Assignment Questions
IGNOU BPHCT-135 (January 2024 – December 2024) Assignment Questions
PART A
1. a) Write the assumptions of kinetic theory of gases. Derive the following expression of the pressure exerted by an ideal gas:
Also, using this expression deduce Avogadro’s law. What is the kinetic interpretation temperature?
b) The expression for the number of molecules in a Maxwellian gas having speeds in the range v to v + dv is given by
Obtain an expression of average speed and root mean square speed of a molecule.
c) Define mean free path of the molecules of a gas. Show that it is equal to under Zeroth order approximation.
d) What is Brownian motion? Write any four characteristics of Brownian motion.
2. a) What are Intensive and extensive variables. Write two examples of each. List the intensive and extensive variables required to specify the thermodynamic systems
(i) paramagnetic solid and (ii) stretched wire.
b) State Zeroth law of thermodynamics. Discuss how this law introduces the concept of temperature. Write parametric as well as exact equation of state for one mole of a real gas and paramagnetic substance.
c) For a pVT – system, show that:
where βT is the isothermal compressibility and α is isobaric coefficient of volume expansion.
d) What is an adiabatic index? Using the first law of thermodynamics, show that, TV-1 = K when one mole of an ideal gas is made to undergo quasi-static adiabatic expansion.
e) Derive an expression for the work done in an isothermal process of an ideal gas.
PART B
3. a) Define efficiency of a Carnot engine. A Carnot engine has an efficiency of 50% when its sink temperature is at 27°C. Calculate the source temperature for increasing its efficiency to 60%.
b) State third law of thermodynamics. Write its mathematical expression. Discuss some important consequences of third law.
c) Write Maxwell’s relations and using these relations derive first and second energy equations.
d) Derive Clausius-Clapeyron equation for two phases to coexist in equilibrium.
e) What is Joule-Thomson effect? Write the mathematical expression of Joule Thomson Coefficient for van der Waals’ gas. What will be the effect on gas if the intermolecular forces are strong?
4. a) Obtain an expression of single particle partition function. Hence, using this expression obtain expressions for entropy and pressure.
b) Define phase space of the system. Draw the phase space for a linear harmonic oscillator.
c) Establish the Boltzmann relation S = kB lnW.
d) Show that Bose derivation of Planck’s law for energy density is given by